Coupled Oscillator Simulator

The mathematical model below was designed to complement a coupled oscillator lab that used an Air Track. An oscillator is a system that periodically changes its energy and can be either mechanical or electronic. Oscillators are coupled when their connection allows them to exchange their energy. In the case of this lab, the spring and cart system is a mechanical system that periodically converts potential energy to kinetic energy, and similarly kinetic to potential. The coupled oscillator model can be applied to many areas of physics such as the interatomic bonds in atoms and the Earth/Moon/Sun orbiting system.

0.00

Count:

Ang. Freq:

Experiment Parameters


Spring Constants

Initial Displacements

Masses

Lab set-up

Original Experiment

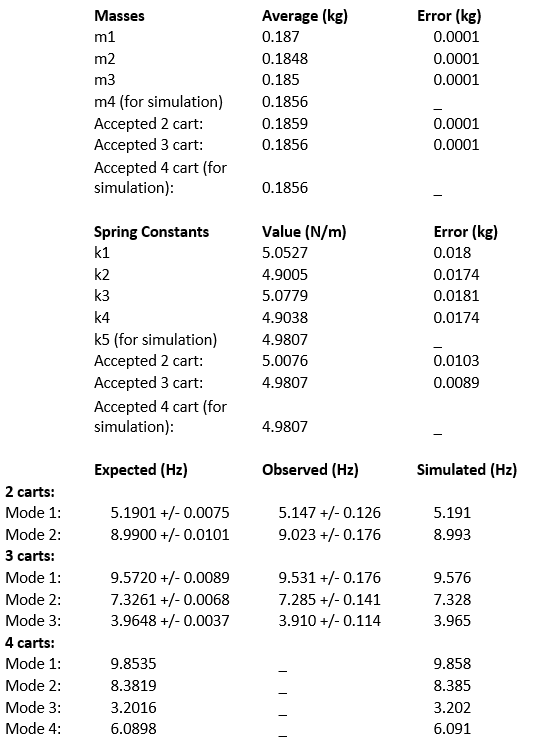
In the original experiment, the fundamental oscillating modes for the 2-cart and 3-cart were studied and the mathematical theory was verified experimentally with the Air Tracks. Fundamental modes provide a basis for all the possible oscillating modes that the system can exhibit.

Theoretical Angular Frequencies

In the table on the right, the theoretical angular frequencies and the required relative amplitudes of the carts is displayed for each of the fundamental oscillating modes. The frequencies for each mode are calculated using “k” (the average spring constant) and “m” (the average mass of the carts). Amplitudes (i.e., Initial Displacement in the simulator) are shown relative to each other. Their exact magnitude is a free choice however the listed relations must stay true.

Experiment Results

The simulated frequency of the fundamental modes compared favorably with the theoretical/expected values with the largest difference being less than 0.005 Hz. See table...